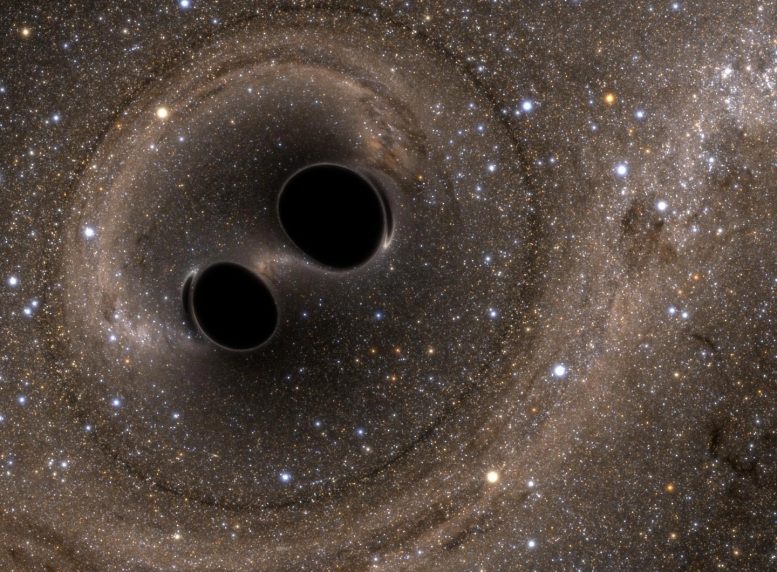
This simulation shows how a black hole merger would appear to our eyes if we could somehow travel in a spaceship for a closer look. It was created by solving equations from Albert Einstein’s general theory of relativity using LIGO data from the event called GW150914. Credit: SXS, the Simulating eXtreme Spacetimes (SXS) project
By listening for specific tones in the gravitational waves of black hole mergers, researchers are putting Albert Einstein’s theories to new tests.
When two black holes collide, they merge into one bigger black hole and ring like a struck bell, sending out ripples in space and time called gravitational waves. Embedded in these gravitational waves are specific frequencies, or tones, which are akin to individual notes in a musical chord.
Now, researchers have detected two such tones for the first time in the “ringdown” of a newly formed black hole. Previously, it was assumed that only a single tone could be measured and that additional tones, called overtones, would be too faint to be detected with today’s technologies.
“Before, it was as if you were trying to match the sound of a chord from a guitar using only a single string,” says Matthew Giesler, a graduate student at Caltech and second author of a new study detailing the results in the September 12 issue of Physical Review Letters. Giesler is the lead author of a related paper submitted to Physical Review X about the technique used to find the overtones.
The results, which were based on reanalyzing data captured by the National Science Foundation’s LIGO (Laser Interferometer Gravitational-wave Observatory), have put Albert Einstein’s general theory of relativity to a new kind of test. Because merging black holes experience crushing gravity, studies of these events allow researchers to test the general theory of relativity under extreme conditions. In this particular case, the researchers tested a specific prediction of general relativity: that black holes can be fully described by just their mass and rate of spin. Yet again, Einstein passed the test.
“This kind of test had been proposed long before the first detection, but everybody expected it would have to wait many years before detectors would be sensitive enough,” says Saul Teukolsky (Ph.D. ’73), the Robinson Professor of Theoretical Astrophysics at Caltech and advisor to Giesler. “This result shows that we can start carrying out the test already with today’s detectors by including the overtones, an unexpected and exciting result.”
LIGO made history in 2015 when it made the first-ever direct detection of gravitational waves, 100 years after Einstein first predicted them. Since then, LIGO and its European-based partner observatory, Virgo, have detected nearly 30 gravitational-wave events, which are being further analyzed. Many of these gravitational waves arose when two black holes collided, sending quivers through space.
“A new black hole forms out of a violent astrophysical process and thus is in an agitated state,” says Maximiliano (Max) Isi (Ph.D. ’18), lead author of the Physical Review Letters study, now at MIT. “However, it quickly sheds this surplus energy in the form of gravitational waves.”
As part of Giesler’s graduate work, he started to investigate whether overtones could be detected in current gravitational-wave data in addition to the main signal, or tone, even though most scientists believed these overtones were too faint. He specifically looked at simulations of LIGO’s first detection of gravitational waves, from a black hole merger event known as GW150914.
This simulation shows how a black hole merger would appear to our eyes if we could somehow travel in a spaceship for a closer look. It was created by solving equations from Albert Einstein’s general theory of relativity using LIGO data from the event called GW150914.
The two merging black holes are each roughly 30 times the mass of the sun, with one slightly larger than the other. Time has been slowed down by a factor of about 100. The event took place 1.3 billion years ago.
The stars appear warped due to the incredibly strong gravity of the black holes. The black holes warp space and time, and this causes light from the stars to curve around the black holes in a process called gravitational lensing. The ring around the black holes, known as an Einstein ring, arises from the light of all the stars in a small region behind the holes, where gravitational lensing has smeared their images into a ring.
Credit: SXS
During the end-phase of the merger, a period of time known as the ringdown, the newly merged black hole is still shaking. Giesler found that the overtones, which are loud but short-lived, are present in an earlier phase of the ringdown than previously had been realized.
“This was a very surprising result. The conventional wisdom was that by the time the remnant black hole had settled down so that any tones could be detected, the overtones would have decayed away almost completely,” says Teukolsky, who is also a professor of physics at Cornell University. “Instead, it turns out that the overtones are detectable before the main tone becomes visible.”
The newfound overtones helped the researchers test the “no hair” theorem for black holes—the idea that there are no other characteristics, or “hairs,” needed to define a black hole other than mass or spin. The new results confirm that the black holes do not have hairs, but scientists suspect that future tests of the theory, in which even more detailed observations are used to probe black hole mergers, may show otherwise.
“Einstein’s theory could break down if there are quantum effects at play,” says Giesler. “Newton’s theory of gravity passes many tests where gravity is weak, but completely fails when it comes to describing gravity at its most extreme, like when it comes to trying to describe merging black holes. Similarly, as we eventually probe the signal from black holes with increasing accuracy, it is possible that even general relativity might someday fail the test.”
Over the next few years, planned upgrades to LIGO and Virgo will make the observatories even more sensitive to gravitational waves, revealing additional hidden tones.
“The bigger and louder an event, the more likely LIGO can pick up these overtones,” says Alan Weinstein, a professor of physics at Caltech and a member of the LIGO Laboratory, who is not associated with this study. “With LIGO’s first detection of gravitational waves, we confirmed predictions made by general relativity. Now, by searching for overtones, and even fainter signals called higher-order modes, we are looking for deeper tests of the theory, and even potential evidence of the theory breaking down.”
Says Isi, “Little by little, black holes will shed their mysteries, revolutionizing our understanding of gravity, space, and time.”
The Physical Review Letters study, titled, “Testing the no-hair theorem with GW150914,” was funded by NASA, LIGO, the National Science Foundation, the Simons Foundation, and the Sherman Fairchild Foundation. Other authors include Will Farr (BS ’03) of the Flatiron Institute and Stony Brook University, and Mark Scheel, a research professor of physics at Caltech.
The study submitted to Physical Review X, titled, “Black hole ringdown: the importance of overtones,” was funded by the Sherman Fairchild Foundation, NSF, LIGO, and Caltech. Other authors include Mark Scheel.
For more on this topic, see Scientists Detect Ringing of a Newborn Black Hole and Einstein’s General Relativity Validated 10 Years Ahead of Schedule.
References:
- “Testing the no-hair theorem with GW150914” by Maximiliano Isi, Matthew Giesler, Will M. Farr, Mark A. Scheel and Saul A. Teukolsky, 8 August 2019, Physical Review Letters.
DOI: 10.1103/PhysRevLett.123.111102 - “Black hole ringdown: the importance of overtones” by Matthew Giesler, Maximiliano Isi, Mark Scheel and Saul Teukolsky, 19 March 2019, Physical Review X.
DOI: 10.1103/PhysRevX.9.041060









Please be aware that there is no need to further verify Einstein’s relativity because it has already been disproved both experimentally and theoretically (see peer-reviewed published papers available free of charge at https://www.researchgate.net/publication/297527784_Challenge_to_the_Special_Theory_of_Relativity and https://www.researchgate.net/publication/297528348_Clock_Time_Is_Absolute_and_Universal ). The fatal mistake of Einstein’s relativity is that it uses Lorentz Transformation to redefine time and space and the newly defined time is no longer the physical time we measure with physical clocks as illustrated in the following:
In classical mechanics, in an inertial reference frame (X, Y, Z, T), time T is the absolute Galilean time and space (X, Y, Z) is the rigid Galilean space, which follows Galilean Transformation when observed from another inertial reference frame (X’, Y’, Z’, T’):
T’ = T
X’ = X – vT
Y’ = Y
Z’ = Z
where v is the speed of the reference reframe (X’, Y’, Z’, T’) relative to the reference frame (X, Y, Z, T). In order to produce a constant speed of light relative to all inertial reference frames, Einstein introduces Lorentz Transformation which is mathematically equivalent to a new definition of time (relativistic time t) and space (relativistic space x, y, z):
In an inertial reference frame with isotropic speed of light, he actually defines time and space as follows:
t = T
x = X
y = Y
z = Z
and in any other inertial reference frame (X’, Y’, Z’, T’) with a velocity v relative to the previous inertial reference frame, he defines time t’ and space (x’, y’, z’) as:
t’ = (1/γ)T’ – (γv/c^2)X’
x’ = γX’
y’ = Y’
z’ = Z’
where
γ = 1/(1 – v^2/c^2)^(1/2)
With this definition of time and space, we can derive the relationship between (x, y, z, t) and (x’, y’, z’, t’) as follows:
t’ = (1/γ)T’ – (γv/c^2)X’
= (1/γ)T – (γv/c^2)(X – vT)
= (1/γ)t – (γv/c^2)(x – vt)
= (1/γ + γv^2/c^2)t – (γv/c^2)x
= γ(1/γ^2+ v^2/c^2)t – (γv/c^2)x
= γ(1-v^2/c^2+ v^2/c^2)t – (γv/c^2)x
= γt – (γv/c^2)x
= γ(t – vx/c^2)
x’ = γX’
= γ(X – vT)
= γ(x – vt)
y’ = Y’
= Y
= y
z’ = Z’
= Z
= z
i.e.
t’ = γ(t – vx/c^2)
x’ = γ(x – vt)
y’ = y
z’ = z
This is Lorentz Transformation between two inertial reference frames. That is, all what special relativity does is just a redefinition of time and space. As the new definition of time and space is just a new coordinate system and should not influence the behavior of any physical process including any clock, like the geometry of a circle which is the same no matter whether you use a Cartesian coordinate system or a cylindrical coordinate system to study it, all the problems of relativity are arisen from the mistake equating relativistic time to the time of a real physical clock. We know the physical time Tp shown on any physical clock is
Tp= Ttf/k
where Tt is the theoretical time, f is the frequency of the clock and k is a reference frame independent calibration constant. In Newton’s mechanics, the theoretical time Tt is the absolute Galilean time T, and f is a reference frame independent constant too. Therefore, we can set
k = f
to make the clock show the theoretical time i.e. the absolute Galilean time T:
Tp= Ttf/k = Tf/f = T
But in special relativity, the theoretical time Tt is the reference frame dependent relativistic time t which makes frequency f a reference frame dependent variable too. When a clock is observed in another inertial reference frame, we have
Tt’ = t’ = γt
f’ = f/γ
Tp’ = Tt’f’/k = t’f’/k = (γt)(f/γ)/k = tf/k = Tp
This means that the physical time Tp won’t change with the change of the inertial reference frame, and is Lorentz invariant and absolute, i.e., a clock still measures the absolute Galilean time in special relativity because the effect of the dilation of relativistic time is always canceled by the decrease of its frequency in a physical clock. The absoluteness of the physical time can be more clearly illustrated by the following thought experiment:
There are a series of vertically standing candles with the same ideal burning rate and moving at different constant horizontal velocities relative to an inertial reference frame of (x, y, z, t) where x, y, z, t are relativistic space coordinates and time. At any moment t of relativistic time, all candles have the same height H in the reference frame of (x, y, z, t) and the height has been calibrated as the physical time. Therefore, we have the simultaneous events measured in both relativistic time t and physical time H in the frame of (x, y, z, t): (Candle1, x1, y1, H, t), (candle2, x2, y2, H, t), … (CandleN, xn, yn, H, t). When these events are observed in anther horizontally moving inertial reference frame (x’, y’, z’, t’), according to special relativity, these events can be obtained through Lorentz Transformation: (Candle1, x’1, y’1, H, t’1), (Candle2, x’2, y’2, H, t’2), … (CandleN, x’n, y’n, H, t’n) where t’1, t’2, … and t’n are relativistic times of the events observed in the frame of (x’, y’, z’, t’). It is seen these events have different relativistic times t’1, t’2, … and t’n after Lorentz Transformation in the frame of (x’, y’, z’, t’), i.e., they are no longer simultaneous measured with relativistic time in the frame of (x’, y’, z’, t’), but the heights of the candles remain the same because the vertical heights here do not experience any Lorentz contraction. Since the heights of the candles are the measures of the physical time, we can see these events still have the same physical time, i.e., they are still simultaneous measured with the physical time. Therefore, the physical time is invariant of inertial reference frames and absolute, which is completely different from relativistic time.
With the above relationship between Galilean space and time and relativistic space and time, we can also get Galilean space and time as functions of relativistic space and time, that is, we can say that Galilean space and time still exists in special relativity. In the inertial reference frame with isotropic speed of light, we have:
T = t
X= x
Y = y
Z = z
In any other inertial reference frame (x’, y’, z’, t’), we have:
T’ = γ(t’ + vx’/c^2)
X’ = x’/γ
Y’ = y’
Z’ = z’
As the real speed of light is measured with a physical clock which is absolute Galilean time, the real speed of light should be defined by Galilean time and space. Now we can use special relativity to prove that the real speed of light is not constant but still follows Newton’s velocity addition formula.
In the moving frame, we have the real speed of light:
C’ = X’/T’ = (x’/γ)/[γ(t + vx/c^2)] = [(x’/t’)/γ^2]/[1 + (v/c^2)(x’/t’)]
Since x’/t’ is the relativistic speed of light which is always a constant c, we have:
C’ = [(x’/t’)/γ^2]/[1 + (v/c^2)(x’/t’)] = [(c)(1 – v^2/c^2)]/[1 + (v/c^2)(c)] = c – v
Since in the inertial reference frame with isotropic speed of light, Galilean time and space is the same as relativistic time and space, we have c = t/x = T/X = C and thus:
C’ = C – v
Therefore, the real speed of light is not constant but still follows Newton’s velocity addition formula, which directly denies the postulate of special relativity that the speed of light is constant relative to all inertial reference frames. You can also find detail mathematical proofs that both time dilation and length contraction are illusions in my peer-reviewed published papers as shown above.
The absoluteness of the physical time has been clearly confirmed by the physical experimental evidence that, after all corrections, all the atomic clocks on the GPS satellites are synchronized not only relative to the ground clocks but also relative to each other (i.e. they are synchronized relative to all reference frames) to show the same absolute physical time, which directly denies the claim of special relativity that clocks can never be synchronized relative to more than one inertial reference frame no matter how you correct them because “time is relative” and “simultaneity can be held only in one inertial reference frame”.
As relativistic time is not the physical time we measure with physical clocks, all what relativity describes is irrelevant to real physical phenomena.