How glass dampens sound: University of Konstanz researchers solve a physics mystery – by rediscovering a discarded theory.
Sometimes the knowledge is already there – it has just been overlooked. For roughly fifty years, the unique vibratory behavior of glass at low temperatures has perplexed physicists.
The reason: Glass carries sound waves and vibrations differently than other solids – it “vibrates differently.” But why?
And how can the propagation of sound in glass be calculated correctly? Two Konstanz physicists, Matthias Fuchs, and Florian Vogel, have now found the solution – by taking up an old model, which was created about 20 years ago and was rejected by experts at the time, and reworking it. Their new view on the old theory has now been published in the journal Physical Review Letters.
Damped Vibrations
If you send sound waves through the glass and measure them very accurately, you will notice a certain damping of the vibrations that is absent in other solids. It has far-reaching consequences for the thermal properties of glass, such as heat transfer and heat capacities. The effect is well known in physics, but until now there was no theoretical model that could describe it correctly – and provide the framework for more complex calculations of sound propagation in glass.
Glasses are disordered solids. Unlike crystalline solids, the particles that make up glass are not regularly arranged. In most solids, the particles sit almost perfectly “lined up”, like building blocks arranged in a precise lattice. When a wavelike vibration is excited in such crystalline solids at low temperatures, the particles pass the vibration on to their neighbors without damping. The vibration runs in a uniform wave without loss, comparable to a la-ola wave in a stadium.
Not so in glass: Its particles are not arranged in a regular lattice but have random positions without stringent order. Oncoming oscillation waves are not carried on in a uniform pattern. Instead, the vibrations arrive at the particles’ random positions and are carried forward in a correspondingly random pattern.
The result is that the uniform wave is broken and disperses into several smaller waves. This dispersion effect causes the damping. Physicist Lord Rayleigh used this mechanism of light scattering by irregularities in the atmosphere to explain the blue color of the sky, which is why this effect is called “Rayleigh damping.”
Rediscovery of a Discarded Model
About 20 years ago, physicists Marc Mezard, Giorgio Parisi (Nobel Prize in Physics 2021), Anthony Zee, and colleagues described these anomalies in glass by a model of oscillations in random positions known as “Euclidean random matrix approach” (ERM).
“A simple model that basically was the solution”, says Matthias Fuchs, professor of soft condensed matter theory at the University of Konstanz. However, the model still had some inconsistencies and was therefore discarded by experts – and fell into oblivion.
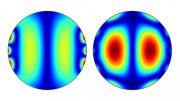
Matthias Fuchs and his colleague Florian Vogel took up the old model again. They found solutions to the open questions the scientific community could not answer at the time and examined the revised model by looking at its Feynman diagrams. These useful graphs were introduced by Richard Feynman in quantum field theory and revealed the regularities in the patterns of the scattered waves.
The results of Matthias Fuchs and Florian Vogel provided true-to-life calculations of the sound propagation and the damping effect in the glass. “Mezard, Parisi, and Zee were correct in their insightful model – harmonic oscillations in a disordered arrangement explain the anomalies of glass at low temperatures,” Fuchs explains.
The re-discovered model, however, is far from the end of the story: “For us, it’s the starting point: We have found the right model that we can now use for further calculations, especially of quantum mechanical effects,” Matthias Fuchs says. “Good vibrations” for research.
Reference: “Vibrational Phenomena in Glasses at Low Temperatures Captured by Field Theory of Disordered Harmonic Oscillators” by Florian Vogel and Matthias Fuchs, 7 June 2023, Physical Review Letters.
DOI: 10.1103/PhysRevLett.130.236101
The research was funded by the German Research Foundation (DFG) in the framework of the Collaborative Research Centre SFB 1432 “Fluctuations and Nonlinearities in Classical and Quantum Matter beyond Equilibrium.”
Never miss a breakthrough: Join the SciTechDaily newsletter.
Follow us on Google and Google News.
4 Comments
According to topological vortex gravitational field theory and uncertainty principle, any physical phenomenon observed in scientific research will not be all of them. “A simple model that basically was the solution”. However, it was discarded by experts – and fell into oblivion. Experts are very serious, and if they cannot observe everything, they will think that the theory is wrong. Therefore, experts can endlessly make assumptions and receive financial support and honor. Experts are really great, and the public is really stupid.
Under the framework of topological vortex gravitational fields, Science and Pseudoscience is clear at a glance. The so-called academic journals and scientific associations should have a better understanding of shame, hypocrisy, and greed than the public.
I think many will require fifty years to figure out the point you are trying to make.
Very correct! If everyone understands, what can experts do?
Well, well. The boys at DARPA will be interested in this development. Their previous research into being able to hear conversations by the vibrations on the exterior glass of rooms could be sharpened by this knowledge. They are pretty generous funding research. Get your funding application in ASAP!