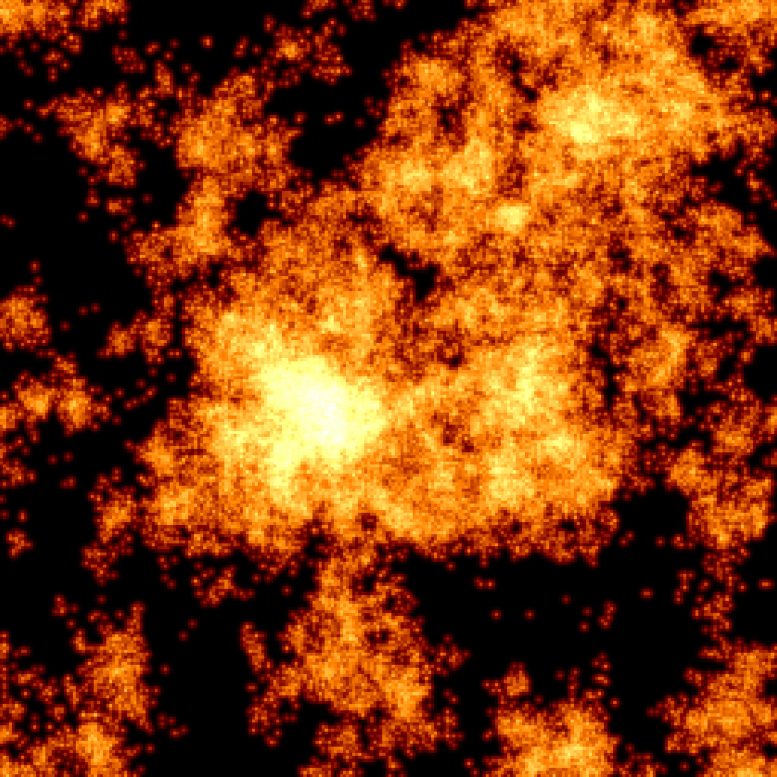
Map of the spatial relaxation in a two-dimensional liquid model. Brighter regions indicate locations where particles moved significantly during some time interval, in dark regions little motion occurred. This image reveals the fractal nature of the relaxation process, shaped both by thermal fluctuations and elastic interactions. Credit: Tahaei et al 2023, Physical Review X (DOI: 10.1103/PhysRevX.13.031034)
Glass is a material that appears simple in its transparency and rigidity but is, in fact, highly complex and intriguing. Its transformation from a liquid to glass, known as the “glass transition,” is marked by a significant slowdown in its dynamics, giving glass its distinctive properties.
This transformation has been a subject of scientific curiosity for years. A particularly interesting aspect of this process is the emergence of “dynamical heterogeneities.” As the liquid cools and nears the glass transition temperature, its dynamics become more correlated and intermittent.
New Theoretical Framework for Glass Formation
In a new study, researchers propose a new theoretical framework to explain these dynamical heterogeneities in glass-forming liquids. The idea is that relaxation in these liquids occurs through local rearrangements, which influence each other via elastic interactions. By investigating the interplay between local rearrangements, elastic interactions, and thermal fluctuations, the researchers have formulated a comprehensive theory for the collective dynamics of these complex systems.
The study is a collaboration between Professor Matthieu Wyart at EPFL and his colleagues at Max Planck Institute in Dresden, the ENS, the Université Grenoble Alpes, and the Center for Systems Biology Dresden. It is now published in Physical Review X.
Scaling Theory and Its Implications
The team developed a “scaling theory” that explains the growth of the dynamical correlation length observed in glass-forming liquids. This correlation length is linked to “thermal avalanches”, which are rare events induced by thermal fluctuations, which then trigger a subsequent burst of faster dynamics.
The study’s theoretical framework also provides insights into the Stoke-Einstein breakdown, a phenomenon where the viscosity of the liquid becomes uncoupled from the diffusion of its particles.
To validate their theoretical predictions, the researchers conducted extensive numerical simulations in various conditions. These simulations supported the accuracy of their scaling theory and its ability to describe the observed dynamics in glass-forming liquids.
Broader Implications and Conclusions
The study not only deepens our understanding of glass dynamics but also suggests a new handle to tackle the properties of some other complex systems where the dynamics are intermittent and jerky- features known to occur in a range of situations, from the brain’s activity or the sliding between frictional objects.
“Our work connects the growth of the dynamical correlation length in liquids to avalanche-type relaxations, well studied, for example, in the context of disordered magnets, granular materials, and earthquakes,” says Matthieu Wyart. “As such, this approach builds unexpected bridges between other fields. Our description of how avalanches are affected by exogeneous fluctuations, including thermal ones, may thus be of more general interest.”
Reference: “Scaling Description of Dynamical Heterogeneity and Avalanches of Relaxation in Glass-Forming Liquids” by Ali Tahaei, Giulio Biroli, Misaki Ozawa, Marko Popović and Matthieu Wyart, 21 September 2023, Physical Review X.
DOI: 10.1103/PhysRevX.13.031034
The study was funded by the Simons Foundation and Schweizerischer Nationalfonds zur Förderung der Wissenschaftlichen Forschung.







According to the theory of topological vortex gravitational field, thermal fluctuations must involve the superposition of topological vortices.