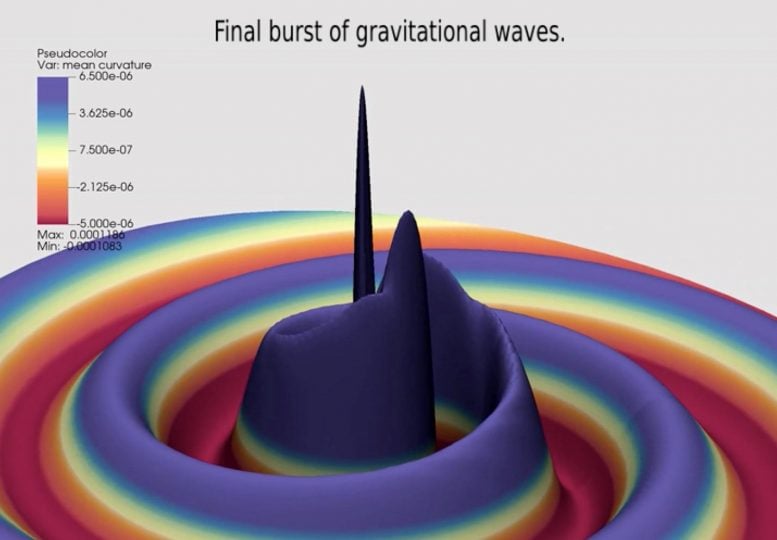
Still from an animation of the inspiral of a binary black hole with a 128:1 mass ratio showing the beginning of the final burst of gravitational waves. Credit: Carlos Lousto, James Healy, RIT
Final Dance of Unequal Black Hole Partners
Rochester Institute of Technology scientists perform first-ever simulation of large mass ratio black hole merger on Frontera.
Solving the equations of general relativity for colliding black holes is no simple matter.
Physicists began using supercomputers to obtain solutions to this famously hard problem back in the 1960s. In 2000, with no solutions in sight, Kip Thorne, 2018 Nobel Laureate and one of the designers of LIGO, famously bet that there would be an observation of gravitational waves before a numerical solution was reached.
He lost that bet when, in 2005, Carlos Lousto, then at The University of Texas at Brownsville, and his team generated a solution using the Lonestar supercomputer at the Texas Advanced Computing Center. (Concurrently, groups at NASA and Caltech derived independent solutions.)
In 2015, when the Laser Interferometer Gravitational-Wave Observatory (LIGO) first observed such waves, Lousto was in shock.
“It took us two weeks to realize this was really from nature and not from inputting our simulation as a test,” said Lousto, now a professor of mathematics at Rochester Institute of Technology (RIT). “The comparison with our simulations was so obvious. You could see with your bare eyes that it was the merger of two black holes.”
Lousto is back again with a new numerical relativity milestone, this time simulating merging black holes where the ratio of the mass of the larger black hole to the smaller one is 128 to 1 — a scientific problem at the very limit of what is computational possible. His secret weapon: the Frontera supercomputer at TACC, the eighth most powerful supercomputer in the world and the fastest at any university.
His research with collaborator James Healy, supported by the National Science Foundation (NSF), was published in Physical Review Letters this week. It may require decades to confirm the results experimentally, but nonetheless it serves as a computational achievement that will help drive the field of astrophysics forward.
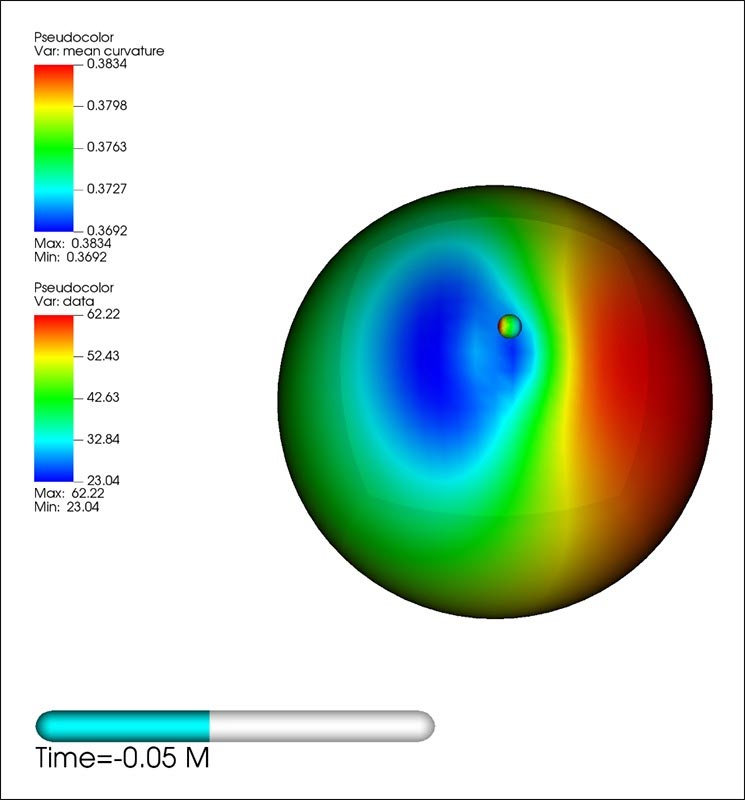
Color map of the curvature on the large black hole horizon generated by the near merging small black hole. Credit: Nicole Rosato
“Modeling pairs of black holes with very different masses is very computational demanding because of the need to maintain accuracy in a wide range of grid resolutions,” said Pedro Marronetti, program director for gravitational physics at NSF. “The RIT group has performed the world’s most advanced simulations in this area, and each of them takes us closer to understanding observations that gravitational-wave detectors will provide in the near future.”
LIGO is only able to detect gravitational waves caused by small and intermediate-mass black holes of roughly equal size. It will take observatories 100 times more sensitive to detect the type of mergers Lousto and Healy have modeled. Their findings show not only what the gravitational waves caused by a 128:1 merger would look like to an observer on Earth, but also characteristics of the ultimate merged black hole including its final mass, spin, and recoil velocity. These led to some surprises.
“These merged black holes can have speeds much larger than previously known,” Lousto said. “They can travel at 5,000 kilometers (3,100 miles) per second. They kick out from a galaxy and wander around the universe. That’s another interesting prediction.”
The researchers also computed the gravitational waveforms — the signal that would be perceived near Earth — for such mergers, including their peak frequency, amplitude, and luminosity. Comparing those values with predictions from existing scientific models, their simulations were within 2 percent of the expected results.
Previously, the largest mass ratio that had ever been solved with high precision was 16 to 1 — eight times less extreme than Lousto’s simulation. The challenge of simulating larger mass ratios is that it requires resolving the dynamics of the interacting systems at additional scales.
Like computer models in many fields, Lousto uses a method called adaptive mesh refinement to get precise models of the dynamics of the interacting black holes. It involves putting the black holes, the space between them, and the distant observer (us) on a grid or mesh, and refining the areas of the mesh with greater detail where it is needed.
Lousto’s team approached the problem with a methodology that he compares to Zeno’s first paradox. By halving and halving the mass ratio while adding internal grid refinement levels, they were able to go from 32:1 black hole mass ratios to 128:1 binary systems that undergo 13 orbits before the merger. On Frontera, it required seven months of constant computation.
“Frontera was the perfect tool for the job,” Lousto said. “Our problem requires high-performance processors, communication, and memory, and Frontera has all three.”
The simulation isn’t the end of the road. Black holes can have a variety of spins and configurations, which impact the amplitude and frequency of the gravitational waves their merger produces. Lousto would like to solve the equations 11 more times to get a good first range of possible “templates” to compare with future detections.
The results will help the designers of future Earth- and space-based gravitational wave detectors plan their instruments. These include advanced, third-generation ground-based gravitational wave detectors and the Laser Interferometer Space Antenna (LISA), which is targeted for launch in the mid-2030s.
The research may also help answer fundamental mysteries about black holes, such as how some can grow so big — millions of times the mass of the Sun.
“Supercomputers help us answer these questions,” Lousto said. “And the problems inspire new research and pass the torch to the next generation of students.”
Reference: “Exploring the Small Mass Ratio Binary Black Hole Merger via Zeno’s Dichotomy Approach” by Carlos O. Lousto and James Healy, 5 November 2020, Physical Review Letters.
DOI: 10.1103/PhysRevLett.125.191102









In a socially distancing earthbound conversation, I can only pray colliding black holes are indicative of the returning King of kings, Jesus.
Irrelevant religious self promotion.
… one more wrong approach, the calculus would not stop at the Plank’s.
What modern physics doesn’t want to understand is that it is not possible to find mathematical formula for every function in the world. In another words, the world is out of mathematical reach. There are way more wrong stuff.
So, next time you try to tell me your physics dogma, there is no time for that, because it should be at true story.
Yes, the truth is out there and you don’t wanna play outside of your little bubbles, because the bubbles tend to burst…
Of course the world is out of mathematical reach, science is based on empiricism and we can’t axiomatize it, c.f. how we can’t do that for quantum field theory.
It is easy for physics to stop before Planck energies, that is precisely that happens in black hole physics – it’s innate to quantum field theory based on observations of Planck’s constant, and applies to gravity.
Why do you reify math? It is just a useful tool for science (and technology), itself useful tool(s) for society. But you can also do math without observation, while observational methodology was that got science started – not math.
““These merged black holes can have speeds much larger than previously known,” Lousto said. “They can travel at 5,000 kilometers per second. They kick out from a galaxy and wander around the universe. That’s another interesting prediction.””
Maybe, but that seems to happen for small black holes at ~ 1/4 mass ratio [their eq. 3 and fig. 4]. It would be odd if galaxies lost super massive central black holes often as galaxies merge and eventually the black holes merge at the center – we don’t seem to see that.
… a weak spot, yes I know what is wrong, and I won’t fix it, because I am not a physicist. Some people just can’t leave it be, as it is, you know.
A good duck sauce! …