
Particle Wave Physics Concept
Complementarity relation of wave-particle duality is analyzed quantitatively with entangled photons as path detectors.
The twenty-first century has undoubtedly been the era of quantum science. Quantum mechanics was born in the early twentieth century and has been used to develop unprecedented technologies which include quantum information, quantum communication, quantum metrology, quantum imaging, and quantum sensing. However, in quantum science, there are still unresolved and even inapprehensible issues like wave-particle duality and complementarity, superposition of wave functions, wave function collapse after quantum measurement, wave function entanglement of the composite wave function, etc.
To test the fundamental principle of wave-particle duality and complementarity quantitatively, a quantum composite system that can be controlled by experimental parameters is needed. So far, there have been several theoretical proposals after Neils Bohr introduced the concept of “complementarity” in 1928, but only a few ideas have been tested experimentally, with them detecting interference patterns with low visibility. Thus, the concept of complementarity and wave-particle duality still remains elusive and has not been fully confirmed experimentally yet.
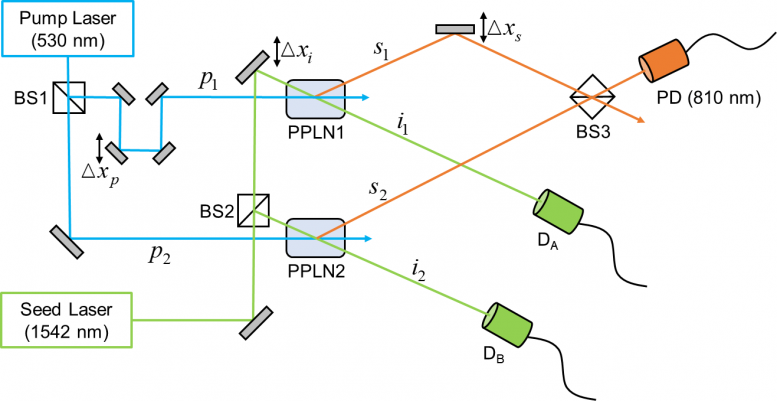
Figure 1. Double-path single-photon interferometer with controllable source purity used in our ENBS model. Two SPDC crystals, PPLN1 and PPLN2, are pumped and seeded simultaneously by the same pump and seed coherent lasers, respectively, resulting in the emission of two signal photons s1 or s2 for quantum interference detection at PD. Then, conjugate idler photons i1 and i2 provide the which-path (or which-source) information, where the controllable source purity is determined by the overlap between the SPACS of one of the idler modes and the unchanged coherent state of another idler mode. Two idler fields can be detected independently by detectors DA and DB. Credit: Institute for Basic Science
To address this issue, a research team from the Institute for Basic Science (IBS, South Korea) constructed a double-path interferometer consisting of two parametric downconversion crystals seeded by coherent idler fields, which is shown in Figure1. The device generates coherent signal photons (quantons) that are used for quantum interference measurement. The quantons then travel down two separate paths before reaching the detector. The conjugate idler fields are used for extracting path information with controllable fidelity, which is useful for quantitatively elucidating the complementarity.
In a real experiment, the source of quantons is not pure due to its entanglement with the remaining degrees of freedom. However, the quanton source purity is tightly bounded by the entanglement between the generated quantons and all the other remaining degrees of freedom by the relation μs = √(1 – E2), which the researchers confirmed experimentally.
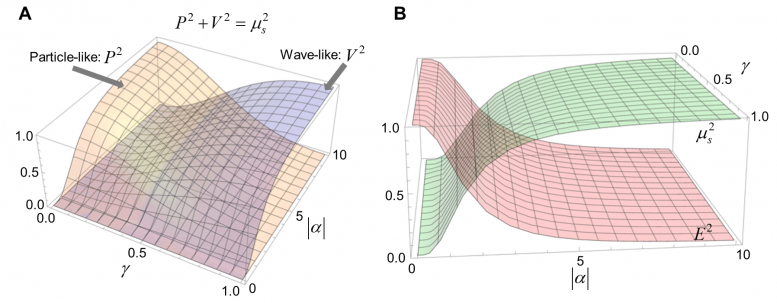
Figure 2. Quantitative complementarity relation of wave-particle duality. (A) Quantitative complementarity relation P2 + V2 = μs2 with respect with respect to γ = ∣α2∣ / ∣α1∣ and ∣α∣ = ∣α2∣. Here, path predictability P represents particle-like behavior, while fringe visibility V represents wave-like behavior of the quanton in the double-path interferometer. The totality of complementarity is bounded by the source purity. (B) Source purity μs of the quanton (signal photon) and entanglement E between the quanton and which-path (which-source) detector form another complementarity relation μs2 + E2 = 1. These two measures are plotted with respect to γ = ∣α2∣ / ∣α1∣ and ∣α∣ = ∣α2∣.
Credit: Institute for Basic Science
The wave-particle duality and the quantitative complementarity P2 + V2 = μs2 (P, a priori predictability; V, visibility) were analyzed and tested using this entangled nonlinear bi-photon source (ENBS) system, where the superposition states of the quantons are quantum mechanically entangled with conjugate idler states in a controllable manner. It was shown that a priori predictability, visibility, entanglement (thus, source purity, and fidelity in our ENBS model) strictly depend on the seed beam photon numbers. This points to the potential application of this approach for the preparation of distant entangled photon states.

Figure 3. Fringe visibility V and a priori visibility V0 as functions of γ = ∣α2∣ / ∣α1∣ and ∣α∣ = ∣α2∣. Blue points are experimental data taken from the team’s recent paper. Experimental data coincide with the visibility V, not a priori visibility V0 across the whole ranges of γ and |α|. This plot validates the team’s analysis of the ENBS experimental results in terms of the wave-particle duality and quantitative complementarity relations. Credit: Institute for Basic Science
Richard Feynman once stated that solving the puzzle of quantum mechanics lies in the understanding of the double-slit experiment. It is anticipated that the interpretation based on the double-path interferometry experiments with ENBS will have fundamental implications for better understanding the principle of complementarity and the wave-particle duality relation quantitatively.
Reference: “Quantitative complementarity of wave-particle duality” by Tai Hyun Yoon and Minhaeng Cho, 18 August 2021, Science Advances.
DOI: 10.1126/sciadv.abi9268








It’s refreshing to see experiments focused on the wave-particle duality, although their concerns about entanglement seem to be getting in the way.
Regarding supersymmetry, one possibility from a view of String Theory is that strings are formed as pairs, one open string matched with one closed string. This may explain some of the curious features we see, such as light being both a particle and a wave. Specifics can be found in the YouTube, Supersymmetry – A String Theory Way at https://www.youtube.com/watch?v=IOndwjeNbjI&t=10s
Spyroe theory claims to solve the wave particle confusion. Double slit paradox solved using spyroe theory.
Quantum theory: quanta exist. Not more, not less. Then, our obsession with waves came about.
The Theorem òf Wave Particle duality is Universal.However Ìnverse Square Law and Ĺambert’s Cosine Law are followed for distance and and angle of plane with soùrce that makes at the point of incedence.
Wave particle duality holds good and is universal obeying all laws of optiçs or physics.So for distance
Inverse Square Law is followed forspecified Intensity.
La mecánica cuántica es la sublimación de la filosofía metafísica e incluso del idealismo alemán anclados a un antropocentrismo arcaico que los hace ser medida de la materia a su imagen y semejanza en sus limitaciones y en la incapacidad de elevar la perspectiva hasta una mentalidad infinita en la que ni las ondas ni las partículas y cualquier tipo de manifestación fenomenológica de la materia, no es otra cosa que, como decía frederick Nietzsche, no pasan de ser interpretaciones que aunque, parezcan muy absolutas, no son más que, insisto, interpretaciones surgidas del mismísimo vientre del ser humano. http://www.rincondelpensadordesanti. de Santi
De Broglie / Bohm pilot wave theory intrigues me more than any other.
A wave isn’t that something is, a wave is what something does…