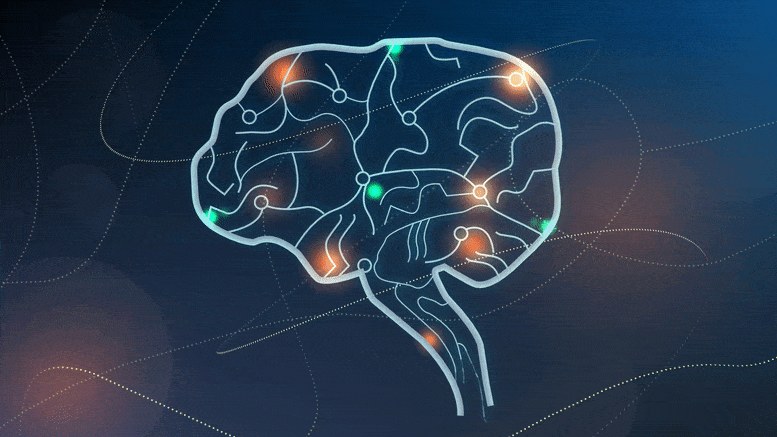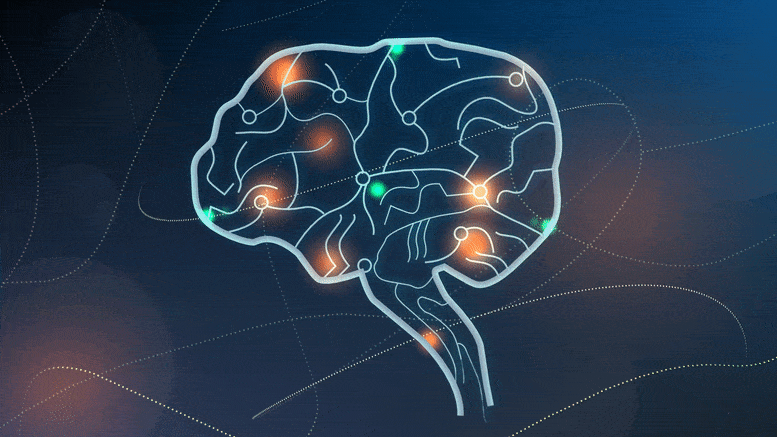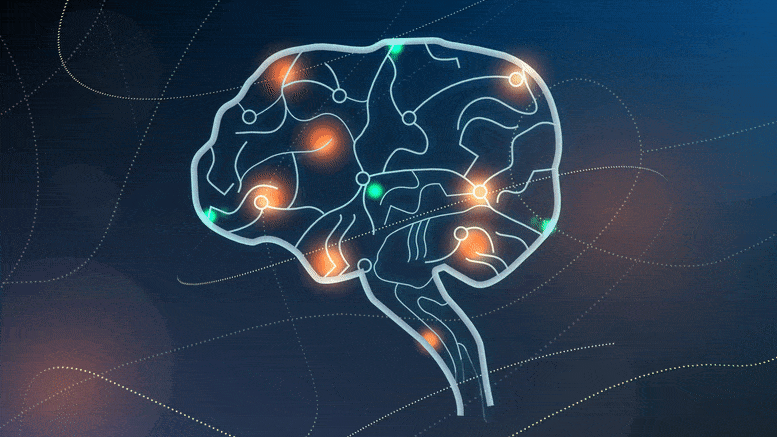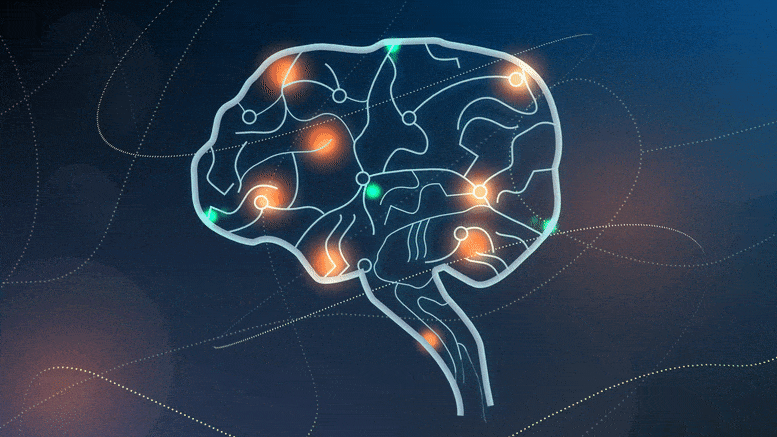
The brain processes math and language in two distinct networks.
Math and language processed by separate networks even if heard together.
Separate math and language networks segregate naturally when listeners pay attention to one type over the other, according to research recently published in JNeurosci.
Mathematical language borrows words from everyday speech, yet the brain processes math and language in two separate networks. While previous studies examined how these networks process written numbers and words, few looked at the processing of spoken mathematical language.
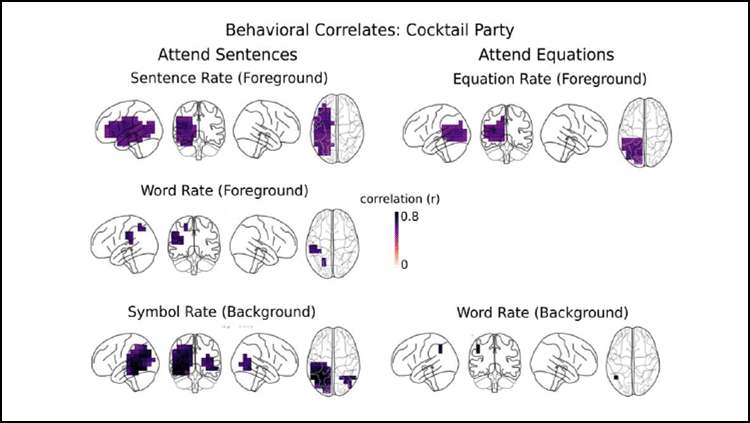
Sentence and equation rate responses are significantly correlated with behavior only if attended, while both attended and unattended word rate responses are significantly correlated with behavior. The sentence rate response is significantly correlated over regions in left temporal, parietal, and frontal areas, while significant correlation for the equation rate response is seen in left parietal and occipital regions. Credit: Kulasingham et al., JNeurosci 2021
Kulasingham et al. used MEG to measure the brain activity of participants while they listened to recordings of simple math equations (five plus one is six) and sentences (cats drink warm milk). In a “cocktail party paradigm,” the equations and sentences played at the same time and participants had to focus on one and ignore the other.
Equations triggered activity in the parietal lobe, an area involved in number processing, and sentences triggered activity in the temporal lobe, a language region. Brain activity oscillated with, or tracked, every word and math symbol, indicating the brain processes their acoustics even if attention was directed away from them. Activity also tracked each sentence or equation — whichever stimulus was focused on — despite the lack of any pauses or inflections to distinguish them from each other.
The results reveal how the brain analyzes the features of different kinds of speech, which may be linked to how we comprehend sentences and calculate equations.
Reference: “Cortical Processing of Arithmetic and Simple Sentences in an Auditory Attention Task” by Joshua P. Kulasingham, Neha H. Joshi, Mohsen Rezaeizadeh and Jonathan Z. Simon, 16 August 2021, JNeurosci.
DOI: 10.1523/JNEUROSCI.0269-21.2021






I just received an important email.
The recognition of division by zero and its implementation on computers will progress rapidly.
This is because they have already learned the division by zero method from the process and have been discussing it.
They are already paying attention to the first issue of the magazine.
2021.7.30. 6:12
Dear Researcher,
I came across your recent publication titled “Geometry and Division by Zero Calculus”, and found your work very interesting:
https://www.researchgate.net/publication/353002859_GEOMETRY_AND_DIVISION_BY_ZERO_CALCULUS
We just released the Notebook Archive, and would like to invite you to make your work available on this site:
https://www.notebookarchive.org
Adding your material in a Wolfram Notebook to the archive would greatly benefit other Mathematica and Wolfram Language users in the future, and broaden the exposure of your work.
Additionally, the notebook will not only be immediately visible but also evaluatable in the Wolfram Cloud.
We hope to develop the Notebook Archive into a broadly useful and popular resource for students and professionals, as we have done with our Demonstrations website:
https://demonstrations.wolfram.com
It will cover a broad spectrum of applications and provide a convenient tool for teaching, with directly accessible and modifiable code.
You can simply upload your notebook (.nb) by going to the following page:
https://www.notebookarchive.org/
You can also send the notebook(s) to me to upload your work on your behalf.
Of course, we encourage you to upload any other notebooks that you think are of relevance.
Sincerely,
Eila Stiegler
The Wolfram Publication Watch Team
Ankur Tiwari’s Great Discovery of the Division by Zero $1/0 = \tan (\pi/2) = 0$ on $ 2011$
Authors: Saburou SaitohYoshinori Saitoh
↓
https://vixra.org/abs/2003.0071
https://vixra.org/pdf/2003.0071v1.pdf
It was shortened to 45 minutes.
↓
https://media.cmd.gunma-u.ac.jp/media/Play/ba02ed49e73949ff84dedcd3e41f76b41d
For Fundamental of Mathematics, I think the division by zero was known as the generalized Moore-Penrose solution of the fundamental equation: ax=b. Please look the simple evidence of its importance: viXra:2010.0228 submitted on 2020-10-28 21:39:06,
Division by Zero Calculus and Euclidean Geometry – Revolution in Euclidean Geometry I gave a simple talk for its essence at some international conference: https://media.cmd.gunma-u.ac.jp/media/Play/ef7ca967c3fd4dabb188128fd6038cb81d
I think our mathematics is not complete still in a serious sense.May I hear your kind comments and suggestions on my idea? Saburou Saitoh2021.1.10.07:55
ゼロ除算の論文、雑誌 最高のダウンロードを 続けている。
Most popular papers in Advances in Linear Algebra & Matrix Theory
Matrices and Division by Zero z/0 = 0
Tsutomu Matsuura, Saburou Saitoh
Abstract | References Full-Text HTML XML Pub. Date: June 14, 2016
DOI: 10.4236/alamt.2016.62007 12,804 Downloads 14,305 Views Citations
On Classes of Matrices with Variants of the Diagonal Dominance Property
Farid O. Farid
Abstract | References Full-Text HTML XML Pub. Date: June 21, 2017
DOI: 10.4236/alamt.2017.72005 6,854 Downloads 14,395 Views Citations
A New Expression for Rhotrix
Abdul Mohammed
Abstract | References Full-Text HTML Pub. Date: June 16, 2014
DOI: 10.4236/alamt.2014.42011 6,379 Downloads 7,818 Views Citations
https://juniperpublishers.com/bboaj/pdf/BBOAJ.MS.ID.555703.pdf
Is It Really Impossible To Divide By Zero?